Titration Contours Of Diprotic Acids
< img
align=” right” elevation= “233” src =” https://mmsphyschem.com/wp-content/uploads/2016/12/titr-9802187.jpg” size= “124” > A diprotic acid is an acid with 2 ionizable hydrogens such that it generates 2 H+ ions for each and every acid particle. Instances of diprotic acids are H2CARBON MONOXIDE3 (carbonic acid), H2C2O4 (oxalic acid), as well as H2SO3 (sulfurous acid). The ionization of a basic diprotic weak acid ionizes in water in 2 actions:
H2A( aq)
< img elevation=" 16" src ="https://mmsphyschem.com/wp-content/uploads/2016/12/darrow-8802098.gif” size=” 33″ > H+( aq)+ HA– (aq) K a1= [H+]
[ HA-]/ [H 2 A] HA-( aq)< img elevation=" 16" src=" https://mmsphyschem.com/wp-content/uploads/2016/12/darrow-8802098.gif" size=" 33" > H+( aq) + A2-( aq) K a2 = [H+] [A2-]/ [HA–]
Due to the fact that H2A has 2 ionizable hydrogens, its titration contour has 2 equivalence factors, as revealed listed below.
< img
elevation
=” 356″ src=” https://mmsphyschem.com/wp-content/uploads/2016/12/diprotc-8748488.gif” size =” 494″ > The formulas for the acid-base response taking place in between H2A as well as NaOH are:
from the starting to the initial equivalence factor:
H2A( aq) + NaOH( aq)
< img elevation=" 14" src=” https://mmsphyschem.com/wp-content/uploads/2016/12/sarrow-8704447.gif “size
=” 45” > NaHA( aq)
+H2O( l) from the very first to the 2nd
equivalence factor:
NaHA(aq)+ NaOH( aq) Na2A( aq) + H2O( l)
and also for the general response: H 2 A( aq)+ 2NaOH( aq) Na 2 A( aq )+ 2H 2 O( l) At the very first equivalence factor, all the H +( aq) from the very first ionization of H 2 A have actually responded with the NaOH. Atthe 2nd equivalence factor, all the H+ (aq )from the 2nd ionization have actually responded with the NaOH. The quantity of NaOH included at the 2nd equivalence factor is two times that
of the very first equivalence factor. It is feasible to figure out the H 2 A acid dissociation constants, Ka1 and also Ka2, making use of the chart listed below.
Ka1 = [H+] [HA–]/ [H2A] ( 1 )
Ka2 = [H+] [A2-]/ [HA–] ( 2 )
< img
elevation= ”
356″
src=” https://mmsphyschem.com/wp-content/uploads/2016/12/diprotic-7554059.gif “size=” 494″ >. On the chart, the red upright line at 5.12 mL is the very first half-titration factor which stands for when half of the H+( aq) ions in the initial ionization have actually been titrated with NaOH. The initial half-titration factor quantity is one-half the quantity of the initial equivalence factor. At the factor, 5.12 mL, [H2A] = [HA–] which can be replaced in Equation (1) over to generate:
Ka1 = [H+] [HA–]/
[HA–] = [H+]Taking the log of both sides returns:
log Ka1 = log [H+]
– log Ka1 = -log [H+]
pKa1 = pH
This suggests that the pH at the very first half-titration factor, 5.12 mL, equates to the pKa1 of H2A. After pKa1 is figured out from the chart, the formula Ka1 = 10– pKa1 can be made use of to figure out the very first dissociation constant for the acid H2A.
Utilizing the very same actions as above, it can be revealed that pKa2 = pH at 15.39 mL of NaOH.
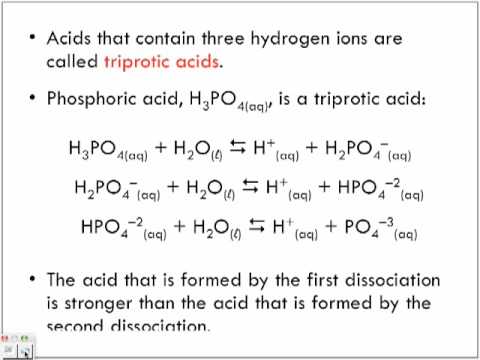
The exact same evaluation can be made use of to identify the Ka‘s of triprotic acids such as phosphoric acid, H3PO4.
House Chart
Leave a Reply